The diagonals of a parallelogram divide it into four triangles of equal area. Consecutive angles are supplementary A D 180.

The Diagonals Of A Parallelogram Bisect Each Other Geometry Help

To answer this question we must find the diagonal of a rectangle that is by Because a rectangle is made up of right angles the diagonal of a rectangle creates a right triangle with two of the sides.

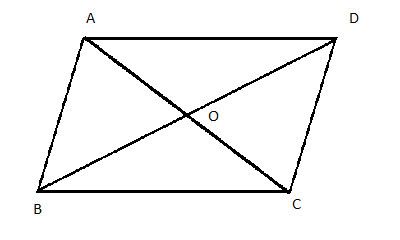
Diagonals of a parallelogram. Diagonals of a parallelogram The use of vectors is very well illustrated by the following rather famous proof that the diagonals of a parallelogram mutually bisect one another. In other words the diagonals intersect each other at the half-way point. And what I want to prove is that its diagonals bisect each other.

These are lines that are intersecting parallel lines. What are the diagonals of a parallelogram. To explore these rules governing the diagonals of a parallelogram use Math Warehouses interactive parallelogram.

Opposite angels are congruent D B. Try this Drag the orange dots on each vertex to reshape the parallelogram. Because the parallelogram has adjacent angles as acute and obtuse the diagonals split the figure into 2 pairs of congruent triangles.

So the first thing that we can think about-- these arent just diagonals. The diagonals bisect each other. The diagonals bisect each other.

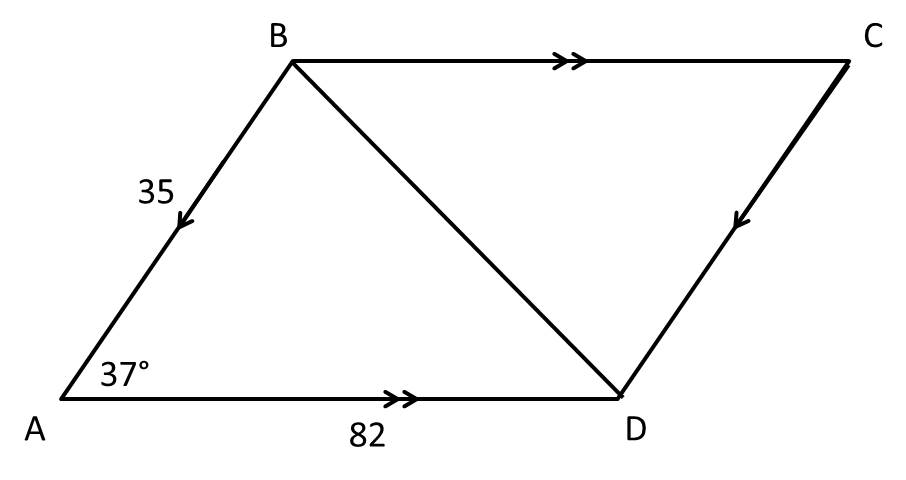
Where pq are the diagonals. Ex 81 2 If the diagonals of a parallelogram are equal then show that it is a rectangle. So if opposite sides of a quadrilateral are parallel then the quadrilateral is a parallelogram.

Proving that a quadrilateral is a parallelogram if and only if its diagonals bisect each otherWatch the next lesson. The diagonals of a parallelogram are equal true or false. 3 Show answers.

Further formulas are specific to parallelograms. Ab are the parallel sides. The diagonals of a parallelogram bisect each other.

Let ABCD be a parallelogram where AC BD To prove. If one angle is right then all angles are right. A parallelogram Suppose that the quadrilateral ABCD in Fig.

The diagonals bisect each other. AO OD CO OB. A parallelogram is a quadrilateral that has opposite sides that are parallel.

So you can also view them as transversals. The diagonals of a parallelogram bisect each other. Ifigured out the question and i dont know what to put here.

All of the area formulas for general convex quadrilaterals apply to parallelograms. Opposite sides are congruent AB DC. There are six important properties of parallelograms to know.

The diagonals of a parallelogram are not equal. A parallelogram has two pairs of equal sides. The diagonals of a parallelogram bisect each other.

That is each diagonal cuts the other into two equal parts. 13 is a parallelogram. So we have a parallelogram right over here.

A parallelogram can be rearranged into a rectangle with the same area. In any parallelogram the diagonals lines linking opposite corners bisect each other. If you just look at a parallelogram the things that look true namely the things on this list are true and are thus properties and the things that dont look like theyre true arent properties.

Since the angles are acute or obtuse two of the shorter sides of the triangles both acute and obtuse are congruent. Diagonals of a parallelogram are the segments which connect the opposite corners of the figure. Diagonals of a parallelogram if you know area angle between the diagonals and other diagonal.

Diagonals of a Parallelogram The diagonals of a parallelogram bisect each other. One pair of opposite sides is parallel and equal in length. It has two pairs of equal angles.

Another question on Mathematics. Notice the behavior of the two diagonals. Parallelograms have opposite interior angles that are congruent and the diagonals of a parallelogram bisect each other.

The opposite sides are parallel. Diagonals of a parallelogram if you know sides and angle or sides and other diagonal- smaller diagonal- larger.