Yes the diagonals of a rectangle bisect each other always. In other words only if the rectangle is a square.

Do The Diagonals Of A Rectangle Bisect The Angles Quora

It has four right.

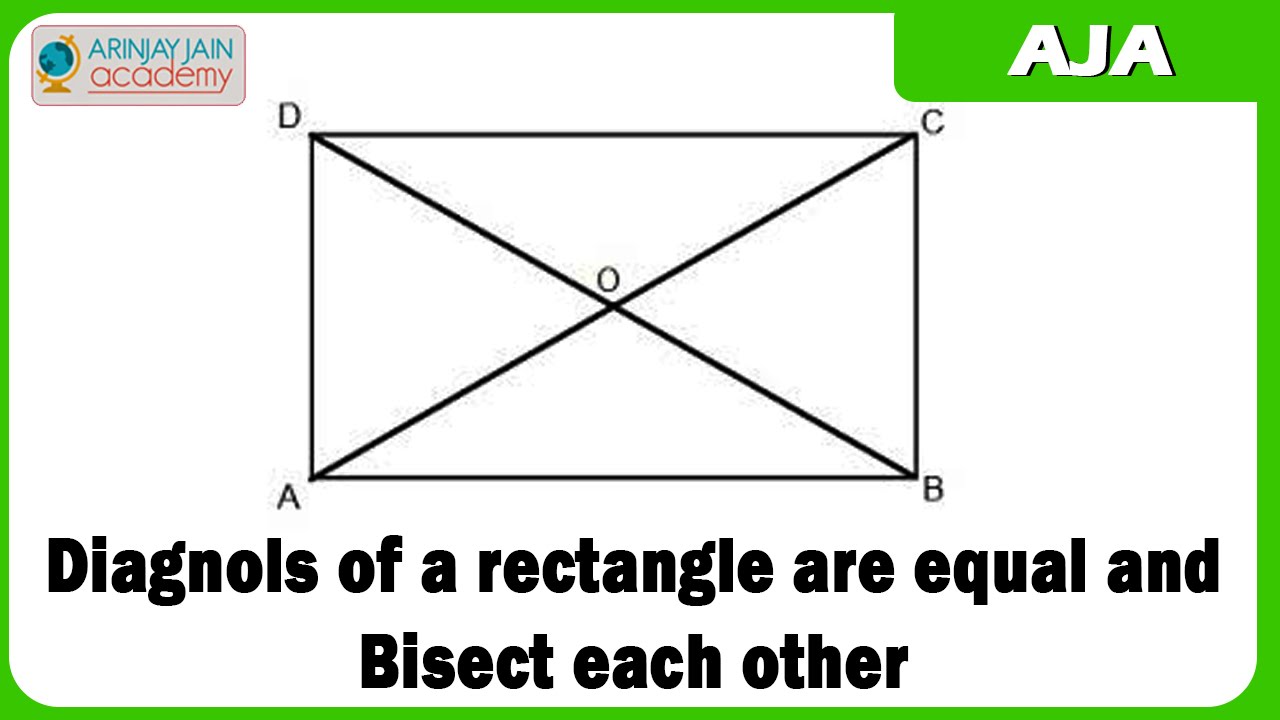
Do the diagonals of a rectangle bisect each other. The square has the following properties. All the properties of a rectangle apply the only one that matters here is diagonals are congruent. Trapezoid since the others fall into the category of the parallelogram whose diagonals always bisect.

Answers Igot 108 hope thats right. And as a square is a special parallelogram which has all the parallelograms basic properties this is true for a square as well. Each diagonal of a rectangle is a diameter of its circumcircle.

The sum of the squares of the sides equals the sum of the squares of the diagonals. The diagonals bisect each other. It has two pairs of equal sides.

Unless the rectangle is a square And the angles formed by the intersection are not always the same. All the properties of a rhombus apply the ones that matter here are parallel sides diagonals are perpendicular bisectors of each other and diagonals bisect the angles. The opposite sides of a rectangle are equal.

Diagonals This page. A rectangle whose side lengths are a and b has area ab sin 90 ab. The opposite sides of a rectangle are parallel.

Each 2 opposite triangles are equals that means the sides are equal. A rectangle has two lines of symmetry. Another way to think of it.

The diagonals of a rectangle will only bisect the angles if the sides that meet at the angle are equal. The opposite sides are parallel. How do you prove that rectangle diagonals bisect each other.

It has rotational symmetry of order two. It has two pairs of equal angles. That is each diagonal cuts the other into two equal parts.

Click here to learn the concepts of Properties of different quadrilaterals from Maths. By drawing the diagonals in a rectangle you form 4 triangles. Diagonals bisect each otherB.

In todays video we will be going over the simple geometry proof that the diagonals of a rectang. Similarly we can prove that PC PA. OP OB.

Another way to think of it. AC and OB are diagonals In the figure let the intersecting point of OB and AC be P To show that diagonals bisect each other we have to prove that OP PB and PA PC The co-ordinates of P is obtained by. In other words only if the rectangle is a square.

The angle is a right- angle and the angle bisector must come out at a half right- angle to the sides. In a square the diagonals bisect each other. The diagonals of a rectangle do not intersect in a right angle they are not perpendicular.

Thus diagonals bisect each other in a rectangle. Read formulas definitions laws from Properties of Different Quadrilaterals here. A rhombus has four sides of equal lengths.

Opposite angles are congruentC. The diagonals of a rectangle bisect each other. In the figure above drag any vertex to reshape the parallelogram and convince your self this is so.

One pair of opposite sides is parallel and equal in length. Adjacent angles are supplementary. Interactive Parallelogram Angles Sides Diagonals The diagonals of a parallelogram bisect each other.

A rectangle whose side lengths are a and b has perimeter 2a 2b. Which of the following is NOT true regarding a rectangle. In any parallelogram the diagonals lines linking opposite corners bisect each other.

This is a general property of any parallelogram. Each other at right angles. The diagonals of a rectangle will only bisect the angles if the sides that meet at the angle are equal.

Each diagonal divides the quadrilateral into two congruent triangles. We have already proven this property for any parallelogram. Diagonals bisect the angles D.

Since the question is about diagonals bisecting each other which effectively means they cut each other in half the correct answer to the question is D. The angle is a right- angle and the angle bisector must come out at a half right- angle to the sides. The diagonals bisect each other at right angles.

Its diagonals bisect with each other. AC and OB are diagonals In the figure let the intersecting point of OB and AC be P To show that diagonals bisect each other we have to prove that OP PB and PA PC The co-ordinates of P is obtained by.

Proof Rectangle Diagonals Bisect Each Other Youtube

Comparison of properties of each type of parallelogram Criteria prove quadrilateral is a parallelogram Menu.

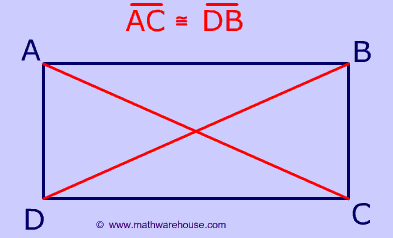
Diagonals of a rectangle bisect each other. The diagonals meet at right angles. The diagonals lines linking opposite corners bisecteach other at right angles 90. OP OB.

The diagonals bisect each other. One of the diagonals bisects the other. It is possible only for square.

That is each diagonal cuts the other into two equal parts and the angle where they cross is always 90 degrees. Answers Igot 108 hope thats right. The opposite sides are parallel.

The sum of the squares of the sides equals the sum of the squares of the diagonals. Prove that diagonals of a rectangle bisect each other and are equalcoordinatebyktcFor Short Notes Revision Notes And NCERT SolutionVisit Us at- wwwkw. If in a parallelogram its diagonals bisect each other right angle and are equal then it is a aSquare bRectangle cRhombus dKite.

Is a rectangle is a parallelogram. About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy Safety How YouTube works Test new features Press Copyright Contact us Creators. The diagonals bisect each other.

How do you prove that rectangle diagonals bisect each other. Adjacent angles are supplementary. Opposite angles are congruentC.

In todays video we will be going over the simple geometry proof that the diagonals of a rectang. No diagonal of rectangle does not bisect each other at right angles. Since the diagonals bisect each other y 16 and x 22.

It is only possible when adjacent sides of rectangle are equal. One pair of opposite sides is parallel and equal in length. Some trapeziums have one line of symmetry.

Vertexto reshape the rhombus and convince your self this is so. Click to see full answer. Diagonals bisect the angles D.

We can prove it by using congruencyWe have to prove that the adjacent triangles in rectangle are congruent. Diagonals bisect each otherB. The length of the mid-segment is equal to 12 the sum of the bases.

Diagonals bisect each other. A rectangle has two pairs of equal sides. A diagonal of a rectangle is inclined to one side of the rectangle at 2 5 o.

Similarly we can prove that PC PA. Which of the following is NOT true regarding a rectangle. Yes since opposite angles are congruent.

Thus a rectangle has all the properties of a parallelogram. A rectangle is a quadrilateral with four right angles. The diagonals of a rectangle bisects each other.

Thus diagonals bisect each other in a rectangle. Each diagonal divides the quadrilateral into two congruent triangles. It has four right angles 90.

Two angles on the same side are supplementary that is the sum of the angles of two adjacent sides is equal to 180. The acute angle between the diagonals is. The acute angle between the diagonals is.

Which statement can be used to prove that a given parallelogram is a rectangleThe opposite angles of the parallelogram are congruentThe consecutive sides of the parallelogram are congruentThe diagonals of the parallelogram bisect each otherThe diagonals of the parallelogram are congruent. A trapezium has one pair of parallel sides. Solution for Properties of Quadrilaterals Diagonals Diagonals Diagonals Diagonals Exactly bisect each other Base Opposite Opposite sldes All angles congruent.

Yes since opposite sides are congruent. Prove by vector method that the quadrilateral whose diagonal bisect each other is a parallelogram.

Proof Diagonals Of A Parallelogram Bisect Each Other Hindi Video Khan Academy

Two angles on the same side are supplementary that is the sum of the angles of two adjacent sides is equal to 180.

Diagonals bisect each other. Its diagonals bisect with each other. The Diagonals of a Square Bisect Each Other Problem. AO OD CO OB.

The opposite sides are parallel. ABCD is a parallelogram diagonals AC and BD intersect at O In triangles AOD and COB DAO BCO alternate interior angles. Also show that they bisect each other at right angles.

A trapezium or a trapezoid is a quadrilateral with a pair of parallel sides. Click to see full answer. Notice the behavior of the two diagonals.

Prove using vectors two lines bisect each other. The length of the mid-segment is equal to 12 the sum of the bases. Thus diagonals bisect each other in a rectangle.

The diagonals bisect each other. Since the diagonals bisect each other y 16 and x 22. Proving that a quadrilateral is a parallelogram if and only if its diagonals bisect each otherWatch the next lesson.

The four small triangles are congruent by SSS. Is a rhombus a parallelogram. Two angles on the same side are supplementary that is the sum of the angles of two adjacent sides is equal to 180.

The diagonals of a parallelogram bisect each other. This means the diagonals form four angles that are congruent and must measure 90 degrees each. The diagonals of a rectangle bisects each other and equal.

Each diagonal divides the quadrilateral into two congruent triangles. If in a parallelogram its diagonals bisect each other right angle and are equal then it is a aSquare bRectangle cRhombus dKite. Show that its diagonals bisect each other that is prove that AOOC and BOOD.

Its diagonals bisect with each other. To explore these rules governing the diagonals of a parallelogram use Math. Adjacent angles are supplementary.

A trapezium or a trapezoid is a quadrilateral with a pair of parallel sides. Diagonals bisect each other. Httpsbitly3akrBoz to get all learning resources as per ICSE CBSE IB Cambridge.

Show Answer x 40 2x 18 40 x 18 40 x 18 22 x. A rhombus has four sides of equal lengths. When we attempt to prove that the diagonals of a square bisect each other we will use congruent triangles.

Solution for Properties of Quadrilaterals Diagonals Diagonals Diagonals Diagonals Exactly bisect each other Base Opposite Opposite sldes All angles congruent. The length of the mid-segment is equal to 12 the sum of the bases. The diagonals of a parallelogram bisect each other.

Theorem 87 If the diagonals of a quadrilateral bisect each other then it is a parallelogram. Trapezoid has two diagonals and both bisect each other. The diagonals bisect each other.

Prove by vector method that the diagonals of a rhombus bisect each other. In any parallelogram the diagonals lines linking opposite corners bisect each other. It has two pairs of equal angles.

Try this Drag the orange dots on each vertex to reshape the parallelogram. What is x and Y. One pair of opposite sides is parallel and equal in length.

Rhombus Square 32 Rhombus Note. ABCD is a square. That is each diagonal cuts the other into two equal parts.

Proof that the diagonals of a. The diagonals bisect each other at right.